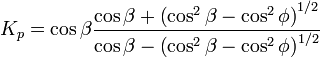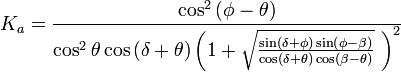The unsaturated zone is the part of the subsurface between the land surface and the groundwater table. The definition of an unsaturated zone is that the water content is below saturation (for the specific soil). Hence, ‘unsaturated’ means that the pore spaces between the soil grain particles or the pore space in cracks and fissures are partially filled with water, partially with air. The unsaturated zone can be from meters to hundred of meters deep.
If an unsaturated zone exists below the ground surface the water infiltrating through the top soil will flow vertically through the unsaturated zone before the water recharges the saturated zone. From the unsaturated zone, the water is lost by i) plant uptake (transpiration), ii) direct soil evaporation and iii) recharge. In the unsaturated zone, the driving force for the flow of water is the vertical gradient of the hydraulic head (consisting of gravity and capillary forces), and the soil characteristics (unsaturated hydraulic conductivity).
The vertical flow through an unsaturated soil is solved numerically using the Richards Equation. This equation is developed by combining the Darcy’s law with the law of conservation of mass and the result is a partial differential equation for one-dimensional vertical flow in unsaturated soil.
An example of lateral earth pressure overturning a retaining wall
Lateral earth pressure is the pressure that soil exerts in the horizontal direction. The lateral earth pressure is important because it affects the consolidation behavior and strength of the soil and because it is considered in the design of geotechnical engineering structures such as retaining walls, basements, tunnels, deep foundations and braced excavations.
The coefficient of lateral earth pressure, K, is defined as the ratio of the horizontal effective stress, σ’h, to the vertical effective stress, σ’v. The effective stress is the intergranular stress calculated by subtracting the pore pressure from the total stress as described in soil mechanics. K for a particular soil deposit is a function of the soil properties and the stress history. The minimum stable value of K is called the active earth pressure coefficient, Ka; the active earth pressure is obtained, for example,when a retaining wall moves away from the soil. The maximum stable value of K is called the passive earth pressure coefficient, Kp; the passive earth pressure would develop, for example against a vertical plow that is pushing soil horizontally. For a level ground deposit with zero lateral strain in the soil, the “at-rest” coefficient of lateral earth pressure, K0 is obtained.
There are many theories for predicting lateral earth pressure; some are empirically based, and some are analytically derived.
At rest lateral earth pressure, represented as K0, is the in situ lateral pressure. It can be measured directly by a dilatometer test (DMT) or a borehole pressuremeter test (PMT). As these are rather expensive tests, empirical relations have been created in order to predict at rest pressure with less involved soil testing, and relate to the angle of shearing resistance. Two of the more commonly used are presented below.
The active state occurs when a retained soil mass is allowed to relax or deform laterally and outward (away from the soil mass) to the point of mobilizing its available full shear resistance (or engaged its shear strength) in trying to resist lateral deformation. That is, the soil is at the point of incipient failure by shearing due to unloading in the lateral direction. It is the minimum theoretical lateral pressure that a given soil mass will exert on a retaining that will move or rotate away from the soil until the soil active state is reached (not necessarily the actual in-service lateral pressure on walls that do not move when subjected to soil lateral pressures higher than the active pressure). The passive state occurs when a soil mass is externally forced laterally and inward (towards the soil mass) to the point of mobilizing its available full shear resistance in trying to resist further lateral deformation. That is, the soil mass is at the point of incipient failure by shearing due to loading in the lateral direction. It is the maximum lateral resistance that a given soil mass can offer to a retaining wall that is being pushed towards the soil mass. That is, the soil is at the point of incipient failure by shearing, but this time due to loading in the lateral direction. Thus active pressure and passive resistance define the minimum lateral pressure and the maximum lateral resistance possible from a given mass of soil.
Rankine theory
Rankine’s theory, developed in 1857,[4] is a stress field solution that predicts active and passive earth pressure. It assumes that the soil is cohesionless, the wall is frictionless, the soil-wall interface is vertical, the failure surface on which the soil moves is planar, and the resultant force is angled parallel to the backfill surface. The equations for active and passive lateral earth pressure coefficients are given below. Note that φ’ is the angle of shearing resistance of the soil and the backfill is inclined at angle β to the horizontal
For the case where β is 0, the above equations simplify to
Coulomb theory
Coulomb (1776)[5] first studied the problem of lateral earth pressures on retaining structures. He used limit equilibrium theory, which considers the failing soil block as a free bodyin order to determine the limiting horizontal earth pressure. The limiting horizontal pressures at failure in extension or compression are used to determine the Ka and Kprespectively. Since the problem is indeterminate,[6] a number of potential failure surfaces must be analysed to identify the critical failure surface (i.e. the surface that produces the maximum or minimum thrust on the wall). Mayniel (1908)[7] later extended Coulomb’s equations to account for wall friction, symbolized by δ. Müller-Breslau (1906)[8] further generalized Mayniel’s equations for a non-horizontal backfill and a non-vertical soil-wall interface (represented by angle θ from the vertical).
Instead of evaluating the above equations or using commercial software applications for this, books of tables for the most common cases can be used. Generally instead of Ka, the horizontal part Kah is tabulated. It is the same as Ka times cos(δ+θ).
The actual earth pressure force Ea is the sum of a part Eag due to the weight of the earth, a part Eap due to extra loads such as traffic, minus a part Eac due to any cohesion present.
Eag is the integral of the pressure over the height of the wall, which equates to Ka times the specific gravity of the earth, times one half the wall height squared.
In the case of a uniform pressure loading on a terrace above a retaining wall, Eap equates to this pressure times Ka times the height of the wall. This applies if the terrace is horizontal or the wall vertical. Otherwise, Eap must be multiplied by cosθ cosβ / cos(θ − β).
Eac is generally assumed to be zero unless a value of cohesion can be maintained permanently.
Eag acts on the wall’s surface at one third of its height from the bottom and at an angle δ relative to a right angle at the wall. Eap acts at the same angle, but at one half the height.
Caquot and Kerisel
In 1948, Albert Caquot (1881–1976) and Jean Kerisel (1908–2005) developed an advanced theory that modified Muller-Breslau’s equations to account for a non-planar rupture surface. They used a logarithmic spiral to represent the rupture surface instead. This modification is extremely important for passive earth pressure where there is soil-wall friction. Mayniel and Muller-Breslau’s equations are unconservative in this situation and are dangerous to apply. For the active pressure coefficient, the logarithmic spiral rupture surface provides a negligible difference compared to Muller-Breslau. These equations are too complex to use, so tables or computers are used instead.
Equivalent fluid pressure
Terzaghi and Peck, in 1948, developed empirical charts for predicting lateral pressures. Only the soil’s classification and backfill slope angle are necessary to use the charts.
Bell’s relationship
For soils with cohesion, Bell developed an analytical solution that uses the square root of the pressure coefficient to predict the cohesion’s contribution to the overall resulting pressure. These equations represent the total lateral earth pressure. The first term represents the non-cohesive contribution and the second term the cohesive contribution. The first equation is for an active situation and the second for passive situations.
Alhamdulillah.. we had initiate our first unsaturated soil mechanics research collaboration between UTM and USM. Thanks for Dr. Ahmad Safuan and Dr. Hetty for initiating the idea & continuous efforts. We love to welcome Dr. Mastura and Dr. Haris for this collaboration. The area of research that we will work together on the Rainfall induce slope. We hope the research will be a smooth sailing. In Sya Allah…
I m looking forward for any colobration in unsaturated soil research framework particularly in ASEAN countries.
Currently we (FKA) had purchase our first unsaturated research apparatus which is 15 bar pressure extractor. Follow with GDS Double Wall Triaxial Cell.
I wish that FKA Geotechnical Research group could further our interest in coupling effect of hydro-mechnanical behaviour of unsaturated soil. Among my future interest on experimental works in Unsaturated as follow:-
Application of variable suction measurement appratus (Osmotic, Axis translation and Filter paper technique)
Application of volume change measurement of unsaturated soil sample using double wall and close range photogrometry or PIV
Investigation of various unsaturated soil types (compacted kaolin, compacted tropical residual soil and peat)
Rainfall induces suction
Early warning system for unsaturated slope stability
Last 40 years, most of soil mechanics researches and practices based on saturated and dry soils mechanics. Unsaturated soil is barely been discussed in details, but last 20 years the development and understanding been gradually increase as there are growing concerns on subject.
An unsaturated soil has commonly been refereed to as a three-phase system containing solid, water and air phases. However, Fredlund & Rahardjo (1993) have postulated that unsaturated soil is a four phase material containing three phases as mentioned above along with the addition of a “contractile skin” which represents the interfacial surface tension between water and air.
The natural process of de-saturation or practices adopted in geotechnical engineering such as placement and compaction of fills are where the possibility of unsaturated soils to occur. Basically, soils become unsaturated in three different types which are evapo-transpiration from the ground surface, compacted soils and gassy soils.
Geotechnical engineering is the branch of civil engineering concerned with the engineering behavior of earth materials. Geotechnical engineering is important in civil engineering, but is also used by military, mining, petroleum, or any other engineering concerned with construction on or in the ground. Geotechnical engineering uses principles of soil mechanics and rock mechanics to investigate subsurface conditions and materials; determine the relevant physical/mechanical and chemical properties of these materials; evaluate stability of natural slopes and man-made soil deposits; assess risks posed by site conditions; design earthworks and structurefoundations; and monitor site conditions, earthwork and foundation construction.[1][2]
A typical geotechnical engineering project begins with a review of project needs to define the required material properties. Then follows a site investigation of soil, rock, fault distribution and bedrock properties on and below an area of interest to determine their engineering properties including how they will interact with, on or in a proposed construction. Site investigations are needed to gain an understanding of the area in or on which the engineering will take place. Investigations can include the assessment of the risk to humans, property and the environment from natural hazards such as earthquakes, landslides, sinkholes, soil liquefaction, debris flows androckfalls.
Ground Improvement refers to a technique that improves the engineering properties of the soil mass treated. Usually, the properties that are modified are shear strength, stiffness and permeability. Ground improvement has developed into a sophisticated tool to support foundations for a wide variety of structures. Properly applied, i.e. after giving due consideration to the nature of the ground being improved and the type and sensitivity of the structures being built, ground improvement often reduces direct costs and saves time.[3]
A geotechnical engineer then determines and designs the type of foundations, earthworks, and/or pavement subgrades required for the intended man-made structures to be built. Foundations are designed and constructed for structures of various sizes such as high-rise buildings, bridges, medium to large commercial buildings, and smaller structures where the soil conditions do not allow code-based design.
The fields of geotechnical engineering and engineering geology are closely related, and have large areas of overlap. However, the field of geotechnical engineering is a specialty of engineering, where the field of engineering geology is a specialty of geology.
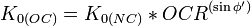
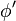is the effective stress friction angle.
